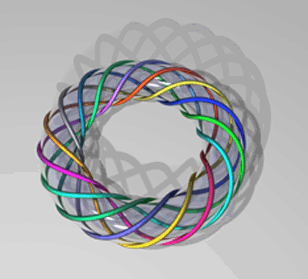
The first section of this part is given to knots: if you make a knot with a rope and then you secure it by joining the two ends, you fix it in something that cannot be untied (unless you made a false knot that by means of manipulation could be reduced to the shape of a circumference). There are simple knots and very complicated ones and the work Continuo infinito presente, by Remo Salvadori, is an example of artistic employment of knots.

First of all the visitor is invited to recognize what are the knots that represent some of the models hung up all along the way. He has got two instruments at his disposal: on a first table there are the same models hung up so that he can hold them and look at them from different points of view, till he recognizes them; on a second table there are some "special" ropes: the visitor can knot them at will and, furthermore, he can join their ends (magnetic) so that each knot can be disposed on a table by flattening it in various positions, each one giving a different way of portraying the same knot.
The same ropes can be also used to reproduce some artistic knots that you can see in Milan (from capitals in Sant' Ambrogio to Borromeo family coat of arms) and that are reproduced on a poster. It is particularly curious to observe that the three rings of Borromeo family coat of arms were not always drawn in the same way and these seemingly "little" changes in the drawing generate completely different knots as a matter of fact.

Another table shows the drawing of a rope carved into a wooden framework; by following the track in the wood it is possible to "make" this knot thanks to available ropes and then fix it by joining the two ends; the result is a knot which looks rather elaborate: if you slip it from the track and you manage it, you can realise how, as a matter of fact, it has a much simpler shape and it is even one of the hung up knots.

Finally two knots are placed in front of a mirror: by means of two available ropes, it is possible to realize that some knots are identical to their mirror image while others are not.

In the second part, the plan of Milan is a good opportunity to propose two classical topological problems.
On a first table there are a good number of maps where some itineraries are marked with different colours: it is a matter of understanding if and how it is possible to go along each itinerary without detaching the pencil from the paper (that is to say, without "flying") and without going along the same street twice (that is to say that you do not want to see things a second time). And trying to understand why some times it is possible to do it and other times it is impossible.

The so-called problem of the "three houses" is shown on a second table: it is a question of linking three landmarks (in our example three stations: Centrale, Nord and Garibaldi) up to three others (in this case: the Duomo, the Linate airport, the Meazza stadium) by means of itineraries that do not intersect. A first map proposes this problem using a "normal" plan of Milan, while a second one let the visitor fly of fancy and presents the same problem in a... science fiction situation: the map is the same but now it is possible to go out from one of its sides, ON CONDITION that you go back into again following some rules (for instance on the opposite side and at the same height) that recall what happens in some video games, when the cursor leaves the screen and reappears on the other side. Some tridimensional models show also how these "rules" are equivalent in the reality to draw the plan of Milan on a surface different from a plane one (a doughnut surface and a Moebius strip surface); an interactive animation proposes the same question on the workstations (from computer screens).

The map of the old Milan with its town walls takes the opportunity to another observation: it is possible to compare the plane map with its transposition on two other exhibited models, a double doughnut and another Moebius strip: of course the distortion is wide but some elements are still clearly recognizable. An animation allows visitors to understand the procedure and the way the map of Milan has been shifted on the double doughnut or on the Moebius strip.